There are consequently 76 possible ways of winning, compared to 10 in a 2D grid. A pretty significant difference, making this game more challenging than it might seem!
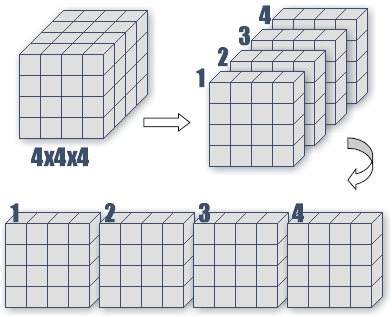
So how is the cube represented in the game? You are shown the four layers of the cube. Imagine the cube was sliced and it's layers placed beside each other. It doesn't matter how the cube is sliced, so long as the layers remain in order.

Frequently Asked Questions
Q: So is this basically four simultaneous games of connect-4?
A: No. Although you can quite happily create lines horizontally, vertically and diagonally on each grid, you must remember that this is a cube and not four individual grids. As well as these possibilities, you can also win by playing through the cube. For example, playing the top-left corner of each layer would create a line.
Q: I understand the theory but could use examples of how to win.
A: Refer to this page: Winning Lines.
Q: Can you reach a stalemate?
A: Yes.
Q: Can you create traps?
A: Yes. There can be a great deal of strategy behind playing 3D 4-in-a-Row, not only can traps be created (a situation where your opponent must block one of your two or more lines), but you can force your opponent to play elsewhere while you create your trap. The more challenging computer opponents employ such tactics.